The musical scale and
its intervals
© 2009
On the meanings of
tones and intervals in the diatonic scale
Temperament, commas,
enharmonics, and other inexactitudes in musical scales
On the meanings of tones and intervals in the diatonic scale
There is a question of long-standing interest concerning how to understand the meaning of the different notes used in music, which convey to listeners such different sensations and feelings.
The frequencies of vibration of the seven notes of the octave of the diatonic major scale, expressed as ratios of integers relative to the frequency of Do are:
C Do – 1/1
D Re – 9/8
E Mi – 5/4
F Fa – 4/3
G So – 3/2
A La – 5/3
B Si – 15/8
In this scale, there are 5 “whole tone” steps, Do-Re, Re-Mi, Fa-So, So-La, La-Si; and two smaller steps called half-tones Mi-Fa and Si-Do (the pattern recommences at the next higher Do whose frequency is two times that of the lower Do).
These are the ratios given in Ouspensky’s In Search of the Miraculous, in the chapter where he discusses this scale as an ancient symbol of esoteric knowledge. Ouspensky, following Gurdjieff[1], calls the half-tone steps the “intervals” of the octave; this differs from the practice in music theory where the movement between any two tones is called an interval.
Many (but not all) other scales, including certain Indian, Chinese, Japanese, and African scales, also have notes whose pitch frequencies can also be given as ratios relative to a root note. A persistent theme in the theory of scales is whether and how the diatonic major scale has some sort of privileged position among scales. This is not entirely a Eurocentric question, because in all of the musical traditions cited, a scale identical to the diatonic occurs and has a place of special importance.
The movements from one tone to another, called “intervals” of music theory, can also be expressed as ratios of pitch. We shall investigate the question of musical meaning from the point of view of the ratios in the notes and intervals of diatonic music. One fact that has long been noted is the different subjective responses to the musical intervals of the 5th (the 5th is defined as an interval incorporating 5 scale steps counting to include the beginning and end notes. Examples would be C-G, A-E, etc.), as opposed to the 3rd’s (there are two types of 3rd’s C-E is a “major third”, A-C is a “minor third”). Also, what is called in music the “inversion of the 5th”, i.e. the 4th (C-F, etc.), has some subjective characteristics related to those of the 5th, perhaps an opposite or complementary characteristic (see below); and an interval of the 6th is subjectively related to the 3rd of which it is an inversion.
Writers such as Danielou and others have offered attempts to rationalize these subjective facts in terms of the prime number factors of the ratios. Here are the prime power factors of the diatonic ratios:
C Do – 1 / 1
D Re – 32 / 23
E Mi – 51 / 22
F Fa – 22 / 31
G So – 31 / 21
A La – 51 / 31
B Si – 3151 / 23
We offer now our own approach to the meaning of musical intervals based on the prime factors. In general terms, the intervals of the 5th and 4th are characterized by the presence of a prime factor of 3, whereas 3rd’s contain the factor 5. What we propose is that a movement between notes whose ratio have the factor 3 in the denominator, for example the 5th’s C-G (3 / 2) and A-E (3 / 4), represents the meaning of a relationship between what is and its representation in mind.
The passage from C to G and back to C, Do–So–Do, is a central feature of music: Schenker and others have observed that this movement underlies a vast range of compositional scales from short melodic phrases up to the whole harmonic arc of a symphony. A similar arc can be found in the tonal music of most cultures that have been studied. In terms of the idea we are proposing, this can be understood as a journey beginning with a doubling of reality by adding the perception of its response in the mind, followed by a return home, enriched by the journey. As T. S. Eliot put it “…the end of all our exploring / Will be to arrive where we started / And know the place for the first time.”
We are encouraged at this point to look at the movement of the 4th. This movement is found in the return path of the Schenkerian arc, the “resolution” of the tension expressed by the 5th. But what about the direct movement of a 4th, say from C to F, Do to Fa, which often occurs as a prelude to the passage from to So, and in other smaller contexts within a musical composition? This movement is felt as a little relaxation which opens up the musical space. Can this be understood as a movement toward something behind reality, toward a more real reality, to which “ordinary” reality has the relation of a dream, or as it is expressed in certain traditions, toward an absolute God (e.g. Brahma) in whose mind reality is dream or concept? Here, Fa sounds as the universal generatrix (see the section “The Mathematics Of Fa”, below, for a mathematical interpretation of this).
We now examine the “circle of fifths”. An old construction of the musical scale going back to Pythagoras (at least) is based on a series of six intervals of a 5th, the ratio 2:3 in frequency, which generates the seven notes of the octave in this order: F C G D A E B. (to put the notes back in order of ascending pitch, one has to then move some of the higher notes down by one, two, or three octaves). This is the classic Pythagorean tuning of the scale, a different tuning than that of the diatonic notes given above. The difference is found first in A, La, which in the Pythagorean tuning is 27/16 in relation to C, whereas the diatonic note is 5/3: the small interval between these two possible A’s, 81/80, is known as the syntonic comma, about a fifth of a half-tone.
Again, as the first note of the circle of 5th’s Fa appears as the generatrix of the Pythagorean scale.
For centuries Pythagorean tuning was used as a practical means for tuning stringed instruments; but it doesn’t produce the pure thirds and sixths of the diatonic scale. However the diatonic scale does not have a pure 5th between D and A, which the Pythagorean does. The difference between this 5th in the two tunings, the “syntonic comma” of about 2 tenths of a semitone, is audible, but it is only a little above the threshold of human perception of difference for successive notes (with simultaneous intervals on the other hand, it is easier to perceive differences, because for example the diatonic 5th D-A has easily detected beats). We note in passing that the equal-tempered (E/T) 5th differs from the Pythagorean 5th by less than 2 hundredths of a semitone, well below the human threshold for perception of difference with successive notes, and even for simultaneous E/T and just 5th’s, the beats are so slow that they are quite difficult to perceive.
What about the interval of the third? Diatonically the major 3rd (C-E) is 5/4, the minor third (A-E for example) is 6/5. Neither interval is exactly represented in E/T; the equal-tempered major 3rd is sharp by 14 hundredths of a semitone and the minor 3rd is flat by slightly more. It is because of this that the system called “just tuning”, with the diatonic ratios, brings a purer sensation, according to some music theorists.
Both major and minor 3rd’s seem to bring in the element of feeling. Let us say then that the prime factor 5 in the major 3rd represents an addition to perception which brings in a feeling about reality; the major third is usually felt as a positive or joyful. The minor 3rd on the other hand, with the factor 3 in the numerator and the factor 5 present as its reciprocal, in the denominator, represents a tripling of perception, with the simultaneous presence of reality, of its representation in the mind, and of the absence of feeling about this duality, or expressed in another way, a receptivity to feeling, an empty space in which feeling can take place and is invited to come into it[2]. The rhetoric of the major 3rd is an expression of joy; the rhetoric of the minor 3rd is that of a question. The inner movement that is indicated in the minor third is an even deeper relaxation than that of the fourth as described above. This gives the minor 3rd its particular character of pathos, so well known in music, and which is indicated in Beelzebub’s Tales as a key to the quality of music that expresses a profound search, and is also so used powerfully in Gurdjieff’s music.
Temperament, commas, enharmonics, and other inexactitudes in musical
scales
Certain writers bemoan the E/T scale as a creation of the Devil, as something that destroys the purity of music and makes true musical expression an absurdity. It appears to us that this is largely a reflex of an attitude that “modernity” represents a fall from a more ancient condition in which human culture was based on something more pure, more true. Historically, modernity developed as a reaction against certain absurdities that accumulated in a European culture that found its sanction in received truth; what happened in reaction to that in turn was the anti-modern attitude. This seems to have been based on two factors: a yearning for a past way of living that seemed better; and certain absurdities that soon began to accumulate in modernity itself (absurdities such as scientism, an overvaluation of the reliability and scope of scientific knowledge).
Since the E/T scale arose within the cultural movements that constituted modernity, and in cultured European musical practice largely replaced earlier scale tunings, it was a natural target for this reaction. We do not agree with the anti-modern reaction in general; and in particular we do not agree with its application to the musical scale.
Studies have shown that skilled performers with instruments having infinitely adjustable pitch, such as voice and violin, only approximately hit the pitches they are aiming at, and the errors are often as large or larger than the difference between the just and the E/T notes; however, listeners do not perceive this as a pitch error. Thus, even if we believe that the just intervals are what the performer is aiming at and that the listener perceives, it would appear that the slightly inaccurate intervals of the E/T scale are close enough to the “just” intervals to awaken a perception of the “just” intervals. The meanings experienced in the just intervals are still felt in music performed in E/T.
This is related to the fact that in human speech the individual speech sounds or “phonemes” are perceived distinctly even though a careful analysis of the frequencies present often reveals substantial differences between what is aimed at and what is actually spoken; evidently the sub-conscious processing in the human auditory system is capable of divining what the speaker or the musician intended and this is what it presents as data for possible conscious apperception, rather than what is actually present in the acoustical vibrations. Indeed, certain brain regions in the left hemisphere are known to subserve perception of sound and meaning in speech, and similar regions, but in the right hemisphere, seem to be involved in the perception of music.
Similar principles apply in all the sensory modalities, and in thinking and feeling: normally, one can become conscious only of what is indicated; not what is actually present. It is true however that special training can interrupt this mechanism; for example in learning to draw, one is taught to ignore the interpretive suggestions from the subconscious and focus on data closer to the actual sensory experience of shape and color, which normally remains sub-conscious. There are important questions, which we will for now not attempt to answer, about when this is worth doing, and to what degree it is possible in a given arena.
We now show diagrammatically (fig. 1, below) a comparison of the E/T and just scales. In the diagram below the circle represents one octave, a pitch ratio from 1 to 2. The outer ticks and their labels show the pitches of the just notes. The equally spaced inner ticks show the pitches of the 12 chromatic E/T notes. The E/T note nearest each of the just notes is the one that would be played on a keyboard instrument. The differences between the E/T and the just notes appear substantial on a visual diagram like this, especially in the case of the Mi, La, and Si; but in terms of actual pitch perception the differences are small, and the ear largely accepts them.
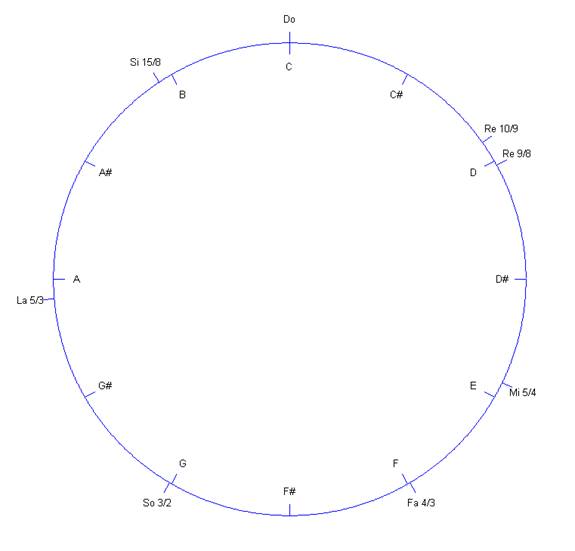
Fig. 1
The equal-tempered and just scales
In fig. 1, we also show a second
candidate for Re whose ratio to Do is 10/9. This is different from the
“standard” Re by a syntonic comma of 80/81. It is important because in the
“minor” or Aeolian scale which consists of the notes La-Si-Do-Re-Mi-Fa-So-La,
Re needs to be a just 4th above the root note
These different versions of notes are all conveniently swept under the rug of equal temperament by regarding all alternate versions of the “same” note as being represented by the nearest E/T note. Sometimes these differences can be notated in standard chromatic notation, where for example F-sharp and G-flat would be represented by the same E/T note—these two differently spelled notes are sometimes called enharmonic, meaning that they are the same E/T note occurring in different harmonic contexts. The two Re’s in fig. 1 are enharmonics that happen to have the same spelling (D) in standard notation.
W. Caryl and colleagues have shown a diagram of overtones of the notes in the fundamental diatonic octave. In this diagram, they are investigating the question of which overtones also occur as overtones of a possibly different note in a higher octave. The conclusion they reach is that the fundamental note Fa “generates” by its harmonics all the notes of the diatonic scale, in the fifth higher octave and above. We shall use mathematical reasoning to prove and explore the conclusions of Caryl.
Let us write the numerators of these fractions as ai and the denominators as bi, so the frequency itself would be ai/bi. Further, for reasons that will be seen, we will factorize each of these integers into powers of primes as follows:
Do i=1 ai=1 bi=1
Re i=2 ai=32
bi=23
Mi i=3 ai=51
bi=22
Fa i=4 ai=22
bi=31
So i=5 ai=31
bi=21
La i=6 ai=51
bi=31
Si i=7 ai=3151
bi=23
We note that the only primes whose powers appear in the diatonic ratios are 2, 3, and 5. Further, the denominators contain only the primes 2 and 3.
A note “diatonically equivalent” to the j-th diatonic note but in the k-th higher octave would be represented as 2k * aj/bj . The question asked by Caryl et. al. is, under what conditions is a harmonic of such a note also a harmonic of a particular note ai/bi in the fundamental diatonic octave. Mathematically expressed, this is the question of when is it true that:
N * ai/bi
= M * 2k * aj/bj (1)
Caryl et. al. communicate a fact they discovered graphically: for the note Fa, but only for the note Fa, it is found that, “eventually”, i.e. for large enough k, all harmonics of (2k * aj/bj) can be found as some harmonic of the note Fa of the fundamental octave, i.e. there is an N such that equation 1 is true. The empirically found minimum value of k for which this is true is 5.
What this might mean has not been clarified by Caryl et. al., but we will offer the following partial interpretation: it is a well-known result of a fundamental theorem due to Fourier that any waveform repeated at a frequency F, which can be regarded as a “musical note” at pitch frequency F, can be represented as a sum (potentially infinite) of sine and cosine waves at frequencies that are harmonics of F, i.e. pure tones of frequencies N * F for all possible N from 1 to infinity. The observation cited above implies that any diatonic musical note of octave k >= 5 can also be expressed as a Fourier sum of certain harmonics of Fa of the fundamental octave; thus, in a certain sense Fa (and Fa only) fully generates by its harmonics all possible diatonic notes.
We call this a partial interpretation because it is not clear to us whether this fact has any consequences that can be directly sensed in the perception of tones. As for what its analogical meaning in the symbolical realm might be, see the section “The Cosmic Role of Fa” below.
We now ask the question: what is the mathematical reason for the observation cited? We want to know, under what conditions can the harmonic M of the note 2k * aj/bj be found also as a harmonic of some fundamental diatonic note ai/bi . By algebraically solving for N, equation 1 can be rewritten as
N = M * 2k * ( aj* bi)/(bj* ai) (2)
The condition that M * 2k * aj/bj is a harmonic of bi/ai is equivalent to saying that N as calculated by equation 2 is an integer. But this can be true only if
M * 2k * ( aj* bi)
contains at least the prime power factors of bj and ai. Now in the case of the fundamental note Fa, ai is 22, so its prime power factors will be contained as long as k >= 2; and since bi is 3, it will cancel the factor 3 that occurs in bj for Fa and La of the higher octave, while for all other higher octave notes the only factors that occur in bj are powers of 2, the highest power being 3 (for Si). Thus, if k >= (3 + 2), all of the prime factors of (bj* ai) will be cancelled, leaving an integer quotient.
To show that Fa is the only fundamental note that has this property, we can reason by cases: for Re, Mi, So, La, and Si, there are prime factors of either 3 or 5 in the numerator (i.e. ai ) which will not be cancelled for those M that do not contain this factor; and in the case of Do there is no factor of 3 in the denominator (bi ) to cancel the factor 3 present in Fa and La of the higher octave.
Q.E.D.[3]
Another way to look at the preceding argument is to consider the Lydian scale, which consists of the notes Fa-So-La-Si-Do-Re-Mi-Fa. Numerically, the pitches of this scale relative to the root note Fa are:
F Fa – 1
G So – 9/8 = 32/23
A La – 5/4 = 51/22
B Si – 45/32 = 3251/25
C Do – 3/2 = 31/21
D Re – 27/16 = 33/24
E Mi – 15/8 = 3151/23
These ratios are the same as for the major scale except for the 6th degree Re, which is the enharmonically equivalent Pythagorean 6th rather than the just 6th; and the 4th degree Si, which is not a just 4th but a different note, an augmented 4th. This note is an example of what was called in the late middle ages “the devil in music.” It is also called the “tritone” because it three whole tones from the root Fa. It is the presence of this note that gives the Lydian mode its unique poignant flavor.
Note that with this change to Fa as root, the only prime whose powers appear in the denominators is 2. Since the highest such power of 2 is 25 the desired conclusion immediately follows, that for all diatonic notes five or more octaves above the fundamental octave, the harmonics of Fa are contained in the harmonics of each note of the higher octave.
Another interesting observation is that the Lydian scale consists of just those ratios subject to four simple rules: only powers of 3 and 5 appear in the numerator; the sum of all the powers of prime in the numerator is at most 3; the power of the factor 5 is at most 1; for all possible numerators that meet these conditions (there are six such), the note is reduced to the fundamental octave, i.e. the appropriate power of 2 is introduced into the denominator. These natural-seeming conditions uniquely determine the Lydian scale. Now, each of the diatonic modal scales including the standard Major scale is a shift of the Lydian scale beginning with a different degree, so the Lydian also uniquely defines the all of these modes. Or does it? Let us look at the ratios of the notes of each mode relative to the root of that mode.
For each note, its diatonic syllable and its ratio to the root of the mode are indicated. A + or – sign is used to indicate that the degree in that mode is augmented or diminished (also called “minor” in the case of 3rd and 6th degrees) relative to its position in the major scale. A * is used to indicate when the ratio is anomalous in a sense that will now be discussed.
|
Mode |
Ionian |
|
Dorian |
|
Phrygian |
|
Lydian |
|
Mixo- |
|
Aeolian |
|
Locrian |
|
|
|
(Major) |
|
|
|
|
|
|
|
Lydian |
|
(Minor) |
|
|
|
|
Degree: |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Root |
Do |
1 |
Re |
1 |
Mi |
1 |
Fa |
1 |
So |
1 |
La |
1 |
Si |
1 |
|
2nd |
Re |
9/8 |
Mi |
10/9 |
Fa - |
16/15 |
So |
9/8 |
La |
10/9 |
Si |
9/8 |
Do - |
16/15 |
|
3rd |
Mi |
5/4 |
Fa *- |
32/27 |
So - |
6/5 |
La |
5/4 |
Si |
5/4 |
Do - |
6/5 |
Re - |
6/5 |
|
4th |
Fa |
4/3 |
So |
4/3 |
La |
4/3 |
Si *+ |
45/32 |
Do |
4/3 |
Re * |
27/20 |
Mi |
4/3 |
|
5th |
So |
3/2 |
La * |
40/27 |
Si |
3/2 |
Do |
3/2 |
Re |
3/2 |
Mi |
3/2 |
Fa *- |
64/45 |
|
6th |
La |
5/3 |
Si |
5/3 |
Do - |
8/5 |
Re * |
27/16 |
Mi |
5/3 |
Fa - |
8/5 |
So - |
8/5 |
|
7th |
Si |
15/8 |
Do - |
16/9 |
Re - |
9/5 |
Mi |
15/8 |
Fa - |
16/9 |
So - |
9/5 |
La - |
16/9 |
Let us look at each of the six “anomalous” notes. Each one requires a correction to make the scale of its mode “normal, ” more harmonious. The 5th of Dorian is calculated as 40/27 which differs by a syntonic comma from the “normal” 5th of 3/2. The 3rd of Dorian, nominally 32/27, also can be corrected by a syntonic comma to the normal minor third 6/5. The 4th of Aeolian is 27/20 which differs, again by a syntonic comma, from a normal 4th of 4/3 (this is the origin of the second Re shown in fig. 1). The 6th of Lydian also differs by a syntonic comma from a normal 6th of 5/3. In order to make the scales and harmonies based on them sound more harmoniously, we would normally adopt these enharmonic alterations whenever we are playing modal music within the given scale.
The augmented 4th of Lydian presents a special problem—it is the tritone, and there are numerous possible ratios that can represent this devilish note. The simplest is 7/5, which differs from 45/32 by a tiny comma of 225/224, a nearly imperceptible difference; but this introduces the new prime factor 7 not previously encountered. Perhaps that is the price of doing business with the Devil. A similar (but inverse) correction applied to the diminished 5th, also a tritone, of the rarely used Locrian mode results in a ratio of 10/7.
In the context of our previous speculations about the subjective meaning of the prime factors 3 and 5, the introduction of 7 raises a new question. Does it perhaps represent a subjective value that is transcendental in comparison to thought and feeling? Such might be the implication of the very special sensations that appear with the 4th degree of Lydian.
The “corrected” modal scale ratios now read like this:
|
Mode |
Ionian |
|
Dorian |
|
Phrygian |
|
Lydian |
|
Mixo- |
|
Aeolian |
|
Locrian |
|
|
|
(Major) |
|
|
|
|
|
|
|
Lydian |
|
(Minor) |
|
|
|
|
Degree: |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Root |
Do |
1 |
Re |
1 |
Mi |
1 |
Fa |
1 |
So |
1 |
La |
1 |
Si |
1 |
|
2nd |
Re |
9/8 |
Mi |
10/9 |
Fa - |
16/15 |
So |
9/8 |
La |
10/9 |
Si |
9/8 |
Do - |
16/15 |
|
3rd |
Mi |
5/4 |
Fa - |
6/5 |
So - |
6/5 |
La |
5/4 |
Si |
5/4 |
Do - |
6/5 |
Re - |
6/5 |
|
4th |
Fa |
4/3 |
So |
4/3 |
La |
4/3 |
Si + |
7/5 |
Do |
4/3 |
Re |
4/3 |
Mi |
4/3 |
|
5th |
So |
3/2 |
La |
3/2 |
Si |
3/2 |
Do |
3/2 |
Re |
3/2 |
Mi |
3/2 |
Fa - |
10/7 |
|
6th |
La |
5/3 |
Si |
5/3 |
Do - |
8/5 |
Re |
5/3 |
Mi |
5/3 |
Fa - |
8/5 |
So - |
8/5 |
|
7th |
Si |
15/8 |
Do - |
16/9 |
Re - |
9/5 |
Mi |
15/8 |
Fa - |
16/9 |
So - |
9/5 |
La - |
16/9 |
Fig. 2 is a circle plot of the degrees of the modal scales. Diminished and augmented degrees are marked by – and +. The anomalous notes have been marked by *; the corrected versions are the nearest numerical degree. The extent to which the actual diatonic ratios of the different degrees cluster around the corresponding E/T notes is made clear by this plot.
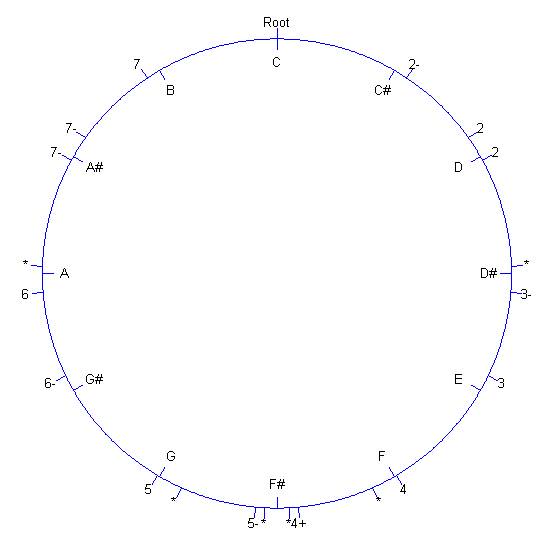
Fig. 2
The degrees of the modal scales
It is worth pointing out that even if, as we have been proposing, Lydian is the architectonic scale which generates all the other modes, after all the generating is done the Lydian itself has to be modified for purposes of harmony, and modified more than any other modal scale.
Note also that a similar construction can be applied to the Pythagorean version of the scale, which consists of six Pythagorean 5th’s starting on Fa, Thus:
Fa=1 Do=3/2 So=9/8 Re=27/16 La=81/64 Mi=243/128 Si=729/512. This is the classic construction often cited justifying the canonical nature of the Lydian and therefore the Major and other modal scales. It is in fact closer to the E/T scale than the just tuning, gradually diverging by about 2/100 of a semitone for each 5th, until the final note in the series Si is about 12/100 semitone sharp, about the same amount that the just Si is flat, relative to E/T. As before, the relative ratios for each mode can then be “corrected” to the nearest “simple” ratios, i.e. ratios involving powers of 2, 3, 5, and 7 with the lowest-value numerator; this yields the same modal scales as before; however the factors used for the corrections are more complex.
We have not yet determined why the Major is to be considered more canonical than the Phrygian or the Mixolydian, the other two modes which escape modification. The only fact that stands out from the table above is that in the Major scale the degrees where it differs from the Phrygian and the Mixolydian have higher frequency ratios. This corresponds to the fact expressed by its name, that the major scale is more “positive” than the other two, which have “minor” versions of the 3rd 6th or 7th degrees. We note in passing that the Lydian, albeit requiring “correction,” is even more “positive” than the Major, having the augmented 4th. Many different proposals have been advanced purporting to show the uniqueness and “inevitability” of the Major diatonic scale, and our reasoning seems about as plausible as any of these.
Exactly what all this mathematics might mean practically is obscure; as has been said, it is difficult to imagine a fact perceptible to sensation that would correspond to the universal generative role of Fa; in addition neither the corrections described above nor the differences between the exact ratio notes and the nearest E/T notes are readily perceived. In practice, almost no musician concerns himself with the exactness of the pitch ratios, but works by an intuition of correct tuning. For this purpose a good enough starting point is the E/T notes closest to the modal notes he is using, and if his performer’s intention is powerful enough to induce in the listener a perception of the precise modal harmonies, well and good.
On the symbolic level, what can be developed from this mathematics is that, yet again, Fa appears as a kind of secret generator of the octave. We might ask ourselves if this has any connection with the “lateral octave” (see fig. 3) described in Ouspensky’s In Search of the Miraculous, which branches off at Fa, and which is presented as the secret key to the purpose of organic life on Earth.

Fig. 3
The Lateral Octave
[In Search of the Miraculous, p. 146]
From another related point of view, in the theory given for the “octave” (Ouspensky, in In Search of the Miraculous) or the “law of seven” (Gurdjieff, in Beelzebub’s Tales) Fa is the note just beyond the first “interval” of the octave. Symbolically, the octave represents any complete process, especially the cosmic process including both the descent of absolute consciousness into matter and the return. The “intervals” in this theory correspond to the two half-tone steps of the scale, and represent points at which an outside force needs to enter a process for it to continue. In the chapter Purgatory in Beelzebub’s Tales, the interval Mi-Fa is the interval in the cosmic octave that was “lengthened” in the process of creation of the present Universe
with the purpose of
providing the ‘requisite inherency’ for receiving, for its [i.e. the
interval’s] functioning, the automatic affluence of all forces which were near…
[Beelzebub’s Tales, first edition, p.
753]
If the extra force that enters at this point is the process of harmonic generation, it may be that this is the very action that we have observed to occur at Fa, which then generates the structure itself of the octave. Further, the 4th degree of the scale on Fa is Si; and it is precisely at this point that the second “interval” in the cosmic octave occurs, the one which was shortened
for the purpose of
facilitating the commencement of a new cycle of its completing process
[Beelzebub’s Tales, first edition, p.
754]
Inasmuch as the Beelzebub of Beelzebub’s Tales was, like the augmented 4th of the Lydian scale, misunderstandingly called “the Devil”, this may be an indication of Beelzebub’s role in our solar system, and hence of Gurdjieff’s.
We will offer one more thought:
that the introduction of the prime factor 7 for the purpose of harmonizing the
scale based on Fa, which was discussed above in the section on “The Mathematics
of Fa”, corresponds to the change in functioning of the law mentioned in
Purgatory. And a meaning of this may be that one of the chief means indicated
for inner development is to introduce the idea of the law of seven into one’s
thinking, and to “try very hard” to understand this law and related laws “of
world creation and world maintenance” [Beelzebub’s
Tales, first edition, p. 755]. And this is one of the things that
Gurdjieff himself tried very hard to accomplish.